|
This section
outlines some information you will need to manually
design your enclosure if you are using a scientific
calculator, or if you are interested in the formulas
involved. You will need the Thiele/Small
specifications for the driver you want to configure,
and at least a rudimentary knowledge of math.
Google, the search-engine, can direct you to a
number of very good free or
low-cost software programs for designing your
enclosure. Theile/Small online Calculator = http://www.webervst.com/spkrcalc/para.htm
Metric to English Multiply English Unit by:
Formulas for geometrical figures pi (π)=3.1415926535 ... Online Calculator: http://www.calculatorsoup.com/
Source:
Spiegel, Murray R.
Mathematical Handbook of Formulas and Tables.
Online EBP Calculator: http://www.calculatoredge.com/new/bandwidth.htm ONLINE CALCULATOR: http://www.diyaudioandvideo.com/Calculator/Box/
These formulas were engineered by D.B. Keele Jr.
using the vented enclosure alignments developed by
A.N. Thiele.
iR = port radius in inches (1/2 diameter)
SOUND VELOCITY ONLINE CALCULATOR: http://www.sengpielaudio.com/calculator-speedsound.htm Sound velocity Density (Rm.temp. air, (Standard) (68° F,dry,no CO2) ft/s cm/s lbs/cu.ft g/cc 1126 33,160 .075 0.001201397774398
EQUATION
FOR A PARABOLA: ONLINE CALCULATOR: http://www.endmemo.com/geometry/parabolaequation.php Definition: ONLINE CALCULATOR: http://www.1728.org/ellipse.htm
Volume: 4/3 times pi times radius cubed. Surface area: 4 times pi times radius squared. Definition:
A Sound Primer on SOUND WAVES IntroductionSound is a vibratory motion of the air. In our ears, it gives the sensation of hearing. It is excited by vibrating bodies and transmitted to our ears without sensible motions of the air. These things have been known since ancient times, but now we can enquire more deeply into what is happening. How fast and how far do the particles of the air move in transmitting the wave? What are the pressure, density and temperature variations in the wave? How much energy is transmitted by the wave? The answers to this question depend on a dynamical theory of sound, which was first worked out by Newton. Plane WavesTo make the problem as simple as possible, without losing any of the important details, let us consider a plane wave of sound. By a plane wave, we mean one in which quantities vary only with the distance along a certain direction, and of course with the time. We also restrict ourselves to sound in a fluid medium, which cannot support shear stresses. The wave motion must be longitudinal, along the direction we have chosen. Let distance along this direction referred to an arbitrary origin be x, so that we can label each particle of the medium by its position x when at rest (we use the word particle in an abstract sense, as if the gas were a continuum without molecular structure, which is a satisfactory approximation in many cases). We further restrict ourselves to propagation in a gas, with a density ρ0 and a pressure p0 when at rest. The wave can be completely described in terms of the displacement ξ(x,t) of the particles originally at any coordinate x at any time t. The time derivative of the displacement is the particle velocity, and the space derivative of the displacement is the dilatation Δ, equal to v - v0/v0, the relative change in unit mass v = 1/ρ. When this is very small compared to unity (as in a normal sound wave), the dilatation is the negative of the condensation s = Δρ/ρ, the relative change in density. Nothing condenses; this is merely a convenient and conventional term. A condensation is part of a sound wave where the density is higher than normal, while in a rarefaction the density is less than normal. Higher density means higher pressure, and lower density lower pressure.
Properties of Sound WavesNow we can write down Newton's Law, and it is seen to be the simple wave equation, whose properties and solutions are so well known. Just obtaining the wave equation says a great deal about sound waves, and lets us apply techniques developed for optics, such as Snell's Law and ray tracing. Under the conditions we have assumed, sound waves travel without dispersion at the phase velocity c = sqrt(γp0/ρ0), and obey the principle of superposition. The conditions for the validity of our analysis are that s << 1, p << p0, u << c, that the gas obeys the ideal gas law, and that γ is constant. For air at 0 °C, p0 = 1 014 000 dyne/cm2, ρ 0 = 0.00129 gm/c3m and γ = 1.41. This gives c = 333 m/s or 1092 ft/s, agreeing well with measurements. Using the ideal gas law, we also find that c = sqrt(γRT/M), where R is the universal gas constant, and M is the molecular weight of the gas. In case the gas cannot be considered ideal, our analysis is still completely valid so long as we use the correct adiabatic compressibility of the gas instead of γp0. In this case, c may not vary exactly as the square root of the absolute temperature. A precise value of c = 331.46 m/s for dry air containing 0.03% CO2 at 0 °C is given in tables, but the actual velocity is affected by vibrational relaxation in O2, the presence of H2O, and other small factors, so the precision is somewhat illusory. At 0 °C the speed of sound in distilled water is 1482 m/s, in sea water of 3.5% salinity 1522 m/s, in mercury 1450 m/s, in steel rails 5050 m/s, and in bulk soft iron 5957 m/s, which should give some idea of the range of phase velocities. One source says the phase velocity in water decreases by 2.4 m/s per °C, though in most liquids it increases with temperature, as in a gas. Another source gives the phase velocity in water as c = 1403 + 4.9t - 0.05t2 + 0.16p m/s, where t is the Celsius temperature, and p is the pressure in bars (106 dy/cm2. This gives an increase with temperature. Harmonic (Sinusoidal) Waves
To get an idea of the magnitudes involved, suppose a loudspeaker cone of 15 cm diameter is radiating 100 W of acoustic power at 1000 Hz. This will be a rather strong sound wave. Now P = 5.6 x 106 erg/s-cm2, so u = 1629 cm/s is the peak particle velocity. The condensation amplitude is s = u/c = 0.05, approximately, which is indeed small compared to unity. The overpressure p = ru = 6940 dy/cm2, and so p/p0 = 0.007, which is satisfactorily small. This is a very conservative estimate, since a normal overpressure is closer to 1 dy/cm2. Note that we have not yet used the frequency, which we need only to find the particle displacement. At 1000 Hz, the wavelength is 33.3 cm, and the wave number k = 0.1887 cm-1, so the particle displacement amplitude is .05 / 0.1887 = 0.265 cm. The ratio ξ/λ = 0.008, so the particle displacement is a very small fraction of a wavelength. The sound pressure level (SPL) in dB is conventionally 20 log (p/2 x 10-5 N/m2), where p is the rms value of the overpressure (peak value divided by the square root of 2). The reference pressure of 20 μPa corresponds to an intensity of 10-12 W/m2, near the threshold of hearing. Velocity PotentialThe description of the motion by the particle displacement ξ as a function of time and the initial position of the particle is very convenient in one-dimensional problems. It is called the Lagrangian picture of fluid motion. An alternative description that is much easier to work with for three-dimensional problems and for deeper theoretical analysis is the Eulerian picture in which the values at a fixed point as a function of time are considered. In this picture, the basic description is in terms of the vector velocity v(x,y,z,t), which is a vector field. The pressure and condensation will be scalar fields, ρ(x,y,z,t) and s(x,y,z,t). The fundamental equations are Newton's law and the equation of continuity, which express the conservation of momentum and mass, respectively. They are most easily expressed in vector calculus notation. Because of browser restrictions, we must use grad, div and curl instead of the del operator. Newton's law is ρDv/Dt = -grad p - ρg, where the last term, the gravitational force, stands for any volume force on the fluid, and D/Dt = ∂/∂t + v·grad is the rate of change operator moving with the fluid. We will omit the gravitational force, and any other constant force, which will have no effect on acoustic disturbances. The equation of continuity is ∂ρ/dρ + div(ρv) = 0. These are the basic equations of hydrodynamics, and are derived in any fluid mechanics text. In sound waves, p and ρ vary little from their steady values (by less than one part in a thousand), and v is much less than the phase velocity of sound. Under these conditions, the equations become very closely linear, and things are very much simpler. For acoustics, the equation of motion is ∂v/∂t = - (grad p)/ρ, and the equation of continuity is ∂ρ/∂t = -ρ div(v) or ∂s/∂t = -div(v). In these equations, ρ is to be taken as a constant, its equilibrium value, and p is the pressure minus the equilibrium pressure. We shall consider the medium to be an ideal fluid of zero viscosity. This has the important consequence that microscopic rotational motion cannot arise if it was initially absent (as is the case when a sound wave passes through the medium). Kelvin stated this in the form (D/Dt)∫v·ds, which says that the circulation around any closed curve remains constant (at zero). This is equivalent to the differential statement curl v = 0, which may be familiar from electrostatics. Just as in electrostatics, this means that we can define a function φ such that v = grad φ (there is no minus sign here, as in electrostatics, as a matter of convention). The function φ(x,y,z,t) is called the vector potential, and allows us to represent the three components of the vector velocity in terms of a single scalar function. If we write the fundamental equations in terms of φ instead of v, we get grad(∂φ/∂t) = -grad(p/ρ) and ∂s/∂t = -div grad φ. The first equation is equivalent to ∂φ/∂t = -(c2/ρ)s, where we have used the compressibility relation p = ρc2s. Now we can eliminate either φ or s between these two equations. Let's eliminate s. The time derivative of the second equation is ∂2φ/∂t2 = -c2∂s/∂t. Using the first equation, we get ∂2φ/∂t2 = c2div grad φ. Recalling that div grad is the sum of the second partials, we recognize this as the three-dimensional wave equation. Let us suppose that φ is known, perhaps as the solution of the wave equation. The other variables are easily expressed in terms of it. First, v = grad φ, from the definition of φ. Then, p = -ρ(∂φ/∂t), and s =-p/ρc2 = -c2(∂φ/∂t). The space derivatives of φ give the velocity, while the time derivative gives the pressure and condensation. The boundary conditions on φ are that φ = 0 at an open end (p = 0), and that ∂φ/∂n = 0 at a closed end (or rigid boundary). Distance normal to the boundary is represented by n ("normal"). For a harmonic plane wave travelling in the direction k, where |k| = k = ω/c, time derivatives are equivalent to multiplication by jω, and grad is equivalent to multiplication by -jk. Then, v = -jkφ, p = -jωρφ and s =-jωc2φ. The wave impedance p/v = ρc is real, so p and v are in phase. The particle velocity v is in the direction of propagation (rather than opposite to it) for a positive condensation. The set-up for the Eulerian picture is more elaborate than for the Langrangian picture, but once we get to the wave equation, everything falls out nicely, and we can treat more complicated problems. The particle velocities will be opposite but equal in the condensations and rarefactions of a propagating wave. If the density were constant, as much air would travel forward in a condensation as would return in the rarefaction. This is the normal case often emphasized in wave motion, where the wave and its energy travel, but the medium does not. However, the density is ρ(1 + s), which is greater in the condensation and less in the rarefaction. Therefore, more air will move forward than returns, though the effect is proportional to sv, the product of small quantities. Strong waves will be associated with mass motion in the direction of propagation. A similar effect occurs for the wave velocity, as a result of the neglected terms in the substantial derivative, making strong waves travel faster than weak ones. These are typical finite-amplitude effects that are interesting to study, but of little practical interest in most cases. AbsorptionOne should distinguish between attenuation, which is a dimunition in intensity for any cause whatever, and absorption, in which energy in the sound wave is transformed into some other form, usually heat. No simple mechanism for the absorption of sound energy by a perfect gas immediately suggests itself, and, in fact, sound does propagate with remarkably little absorption. It is attenuated mainly by spreading, scattering, and absorption by surfaces. The finite viscosity, heat conduction, and molecular mean free path of the medium do give rise to an absorption that increases at higher frequencies. One interesting effect is that the temperature changes in the adiabatic processes may equilibrate with molecular internal degrees of freedom at low frequencies, while at higher frequencies equilibration does not occur. The result is an effective change in γ, which not only changes the phase velocity, but also introduces an absorption because of the phase lag that is effective in the band of frequencies where the phase velocity is changing. The main other contribution to absorption is called viscothermal because it involves these transport properties of the gas, and increases as the square of the frequency. It is also called classical absorption. The distance at which the amplitude of a sound wave is diminished by a factor of 1/e by viscous absorption is (3c/8π2ν)λ2, where ν is the kinematic viscosity, 0.132 cm2/s for air. At 1000 Hz, this distance is more than 10 km. The classical intensity absorption coefficient for air is given in tables as α = 1.61 x 10-10f2 dB/m, where ln(I0/Id) = 2αd. At low pressures, absorption occurs when the wavelength becomes comparable to the molecular mean free path (about 66nm in air at STP). Most of these effects are considerable only at frequencies well above the audible range or at very low pressures. If the mean free path is taken as inversely proportional to the pressure, then the mean free path becomes 1/10 of the wavelength for 1000 Hz at a pressure of about 1.3 μHg, a low vacuum. The effect of humidity on sound propagation is small, but rather complicated. At 68°F, the phase velocity increases from about 1127 ft/s for dry air to about 1131 ft/s at 100% humidity, mainly due to the decrease in density. The absorption at 100 Hz is 1.67 dB/km for dry air, 0.38 dB/km at 50% humidity, and 0.22 dB/km at 100% humidity. At 2000 Hz, the figures are 4.14, 7.14 and 6.29 dB/km. These figures are much larger than the classical absorption calculated from the equation in the preceding paragraph, and are probably due to vibrational and rotational relaxation in water vapour, oxygen, and carbon dioxide. Although absorption in gases is well accounted for by viscothermal and relaxation effects (observed absorption only slightly higher than predicted), absorption in some liquids, such as water or alcohols, is much higher than would be expected on these grounds. The exess absorption can be explained as due to a structural relaxation, a change in the molecular arrangement, during the passage of the wave. Fine wires and threads offer little resistance to the passage of sound. Tyndall found that a piece of felt half an inch thick stopped sound less well than a wet pocket handkerchief. In the latter case, the water closed the pores in the cloth so that it acted like a solid sheet, while the felt did not. This is the reason a hedge is a poor sound barrier, but a tight fence is a much more satisfactory one. Rain and fogs similarly have little effect on sound. In fact, the calmness often found in fogs may actually improve the transmission of sound. However, the presence of moisture catalyzes the absorption by vibrational relaxation in oxygen. Sound OutdoorsIn spite of small absorption, sound cannot be heard for any great distance outdoors. One reason for this is that the temperature of the atmosphere decreases rapidly with altitude (roughly 6 °C for each 1000 m). The lower phase velocity at altitude means that an initially vertical wave front will be tilted backwards, so the rays of sound are bent upwards, creating a shadow at ground level. A strong temperature inversion, or a wind blowing from the source of sound towards the observer, will have the opposite effect, and sound may be heard at a considerable distance. What is important in the case of the wind is that the wind speed generally increases with altitude, a wind shear, not simply a uniform wind. We will consider the effect of the wind in detail below. The unpredictability of the range of foghorns is well known, and can be largely ascribed to such effects. Fog and rain have little effect, since the scale of the disturbances is much smaller than a wavelength. Raising the source of sound has the effect of increasing its range; bells in church towers take advantage of this. A strange effect was long noticed in the audibility of very large explosions and similar noises. The sound is observed in a region surrounding the source, perhaps extending 50 km or more. Then there is a zone of silence, but at several hundred kilometres, the sound, or at least its lower-frequency components, is sometimes again heard, and with extra delay. Guns on the continent were heard in England, for example. In the 1930's, this anomalous propagation was finally recognized as the effect of temperatures comparable to those on the ground, far above the stratosphere. The sound was reflected by these hot layers and again bent downward towards the earth. Sound from sources like jet aircraft or rockets can also be trapped as in a waveguide in the stratosphere, with higher temperatures both above and below, and can be detected thousands of miles from its source by a receiver in the stratosphere.
There are also low-frequency disturbances, well below the range of hearing, that can be detected with a microbarograph or hot-wire microphone, caused by various phenomena around the earth such as volcanoes, earthquakes, ocean waves and so forth. These include the strongly dispersive acoustic gravity waves, trapped in the stratosphere, which are more hydrodynamic phenomena than acoustic ones, that can propagate right around the earth. These disturbances have periods of up to minutes. A flight of stairs may reflect an acoustic impulse in the form of a musical tone. For reinforcement, the depth of the tread of the stairs should be a half wavelength. Since this depth is about 22 cm, the tone should have a frequency of 769 Hz, about a g" (second g above middle C). Rayleigh remarked that a flight of stairs on his estate of Terling returned a handclap as a sound resembling the chirp of a bird. The best situation for observing this effect would be a circular flight of stairs, with the observer standing at the centre of curvature so the sound will be reflected back. So that the delay between the clap and the return will be enough that the return will be easily detected, say about 0.1 s, the distance to the steps and back should be about 33 m, or 100 ft, so the radius of the steps should be about 50 ft. I cannot get the result from an ordinary flight of stairs in a house, probably because one must be too close for the echo to be distinguished. The Doppler Effect
A ratio of frequencies of the twelfth root of two (1.0595), or a semitone, results from a moving source approaching a stationary observer at about 42 mph, which was easily available to Doppler. A full tone difference is attained at 82 mph, and a minor third at 125 mph. A major third is not reached until 150 mph. The speed of sound is taken as 750 mph in these calculations. If the observer were moving at twice the speed of sound away from an orchestra, the music would be heard in the correct tune and time, but backwards, as Rayleigh remarked. Very Strong Sound Waves
Reflection and Refraction
A dense, rigid medium has a very large acoustic impedance. In this case, ξ' = -ξ, and ξ" = 0, so the wave is completely reflected. If the second medium has a much smaller acoustic impedance, the reflected wave is in phase instead. Although actual materials cannot be perfect reflectors, the impedance match between air and a solid or liquid is generally so poor that very little energy goes into sound waves in the denser medium. The behaviour at a solid is quite complicated, because three modes of waves exist in a solid, not just the longitudinal compression wave. Waves from the air do not penetrate into water, and sound waves in water do not penetrate into the air. The two media are acoustically separate. This may be of interest to anglers, who can feel free to talk while they fish. They should beware of casting moving shadows on the water, however. Reflection and refraction in three dimensions is only a little more complicated. Many texts complicate the matter, but it is really easy to understand if you look at it in terms of fundamentals, and not just as a mathematical exercise. The nature of the interface between the media deserves some consideration first. We'll consider two fluid media with a plane interface of a thickness much less than a wavelength. Ideally, we should think of a massless, flexible film separating the two media. When the two media are gaseous, diffusion at least will blur the transition, so such a film would be necessary. The interface between a gas and a liquid, or between two immisicble liquids, would generally suit our condition quite naturally. The wave quantities will be assumed to vary harmonically in time as ejωt. We shall further restrict the analysis to plane waves, which vary harmonically in space according to e-jk·r. The wave vector k points in the direction of propagation, and is of magnitude k = ω/c. The wavefronts, or surfaces of constant phase, are the planes k·r = d + ct. Pressure and particle velocity are functions varying like this, and if p and v are their peak magnitudes (phasors), then v = p/ρc, where ρc is the wave impedance that we defined above. Its units are g/s-cm2 in the cgs system. Its value for air at STP is 43 g/s-cm2. For a positive p, v is in the direction of propagation. Now let's suppose an incident plane wave of amplitude A strikes the interface. The intersection of two planes is a straight line, which we shall call the trace of the wavefront on the interface. As the wavefront moves forward with velocity c, the trace will move parallel to itself with the trace velocity vt (see figure below). In a plane normal to the trace, both the interface and the wavefront will appear as straight lines, and the wavefront will make an angle θ with the interface, called the angle of incidence. A normal to the wavefront, or wave normal, will make the same angle with a normal to the interface. It is now easy to see that the trace velocity is c/sin θ, always greater than or equal to the phase velocity, and infinite for normal incidence. The wavefront does not penetrate the interface, but is replaced on the other side by a refracted wavefront of amplitude C, moving with phase velocity c'. This wavefront must maintain a constant phase relationship with the incident wavefront, and so they have the same trace and, therefore, the same trace velocity. Then, c/sin θ = c'/sin θ', which gives the angle of refraction θ' in terms of θ, c and c'. This is the law of refraction, or Snell's Law. From our construction, we see that the incident and refracted wave normals, and the normal to the interface, all lie in the same plane, the plane of incidence. It was exceedingly difficult to discover this general law empirically, and it was only recognized properly as late as the 17th century. However, we see how easily and naturally it follows from a wave model, and could be extended from acoustics to optics by analogy. It is found that there is generally a third wave of amplitude B, reflected back into the first medium. Just as in the case of the refracted wave, the wavefront must also have a constant phase relation to the incident wave, and so its trace must be the same as the traces of the incident and refracted waves, and move with the same trace velocity. This implies that c/sin θ = c/sin θ", where θ" is the angle of reflection, so that θ" = θ, the familiar law of reflection. Of course, the reflected wave normal also lies in the plane of incidence. The laws of refraction and reflection depend only on the constancy of trace velocity, and so are valid within rather wide limits, and may hold well even when our theoretical relations between the amplitudes do not (as for a thick, but laterally uniform, interface).
After a unit time interval, the trace of the wavefront will have moved from O to Q with velocity vt, while a point originally on the wavefront at P will have moved with velocity c to Q. Therefore, c = vt cos θ, as asserted above. At a unit time interval earlier, the traces were at point R, from which points on the reflected and refracted wavefronts have moved as shown. For an ideal plane interface, the relations between the amplitudes A, B and C are easily found. At the interface, the pressure due to A and B on one side must be equal to the pressure due to C on the other. If this were not true, there would be a finite force on an element of the interface of zero mass, producing an infinite acceleration, which is insupportable. At the point x = 0 and y = 0 this gives A + B = C, and the equality of pressures is guaranteed to hold everywhere on the interface and for any time. Under the action of the wave, the interface must move normally to the surface, in the x-direction. We assume the medium has zero viscosity, so the fluid can slide at will in the y and z directions. We require, therefore, that the normal velocities on each side of the interface be equal, so a gap is not left. The incident pressure wave A creates a velocity v = A/ρc along the wave normal. The x-component of this velocity is A(cos θ/ρc). The reflected wave contributes an x-component -B(cos θ/ρc). The sum of these is the net x-velocity on the left of the interface. On the right-hand side, we have just C(cos θ'/ρc'). Therefore, the boundary condition of equal velocity x-components is (A - B)(cos θ/ρc) = C(cos θ'/ρc'). If we compare this with the similar relations for normal incidence that we obtained at the beginning of this section, we see that they are exactly the same, if we replace ρc by ρc/cos θ, an equivalent "angular" wave impedance that varies from ρc for normal incidence to infinity for grazing incidence. The final results are B = [(ρ'c'/cos θ' - ρc/cos θ)/(ρ'c'/cos θ' + ρc/cos θ)] A, and C = [(2ρ'c'/cos θ')/(ρ'c'/cos θ' + ρc/cos θ)] A, which are very similar to the expressions for normal incidence, if the angular wave impedances are used. In considering energy relations, it must be remembered that when a finite beam of width a is refracted, the refracted beam is of width b = a(cos θ'/cos θ). Reflected and incident beams are, of course, of the same width. If ρ'c' is less than ρc, θ' will reach 90° for some angle of incidence θc less than 90°. Then we will have |B| = |A| for this and larger angles of incidence, and all the incident energy will be reflected. There will, in general, be a phase difference between A and B. C will not be zero, but it will be the amplitude of a boundary, or evanescent wave that carries no energy normal to the interface, but is exponentially attenuated in the second medium. It does carry energy along the boundary, which can have some interesting effects for finite beams. This phenomenon is called total internal reflection, and is studied in optics. For air and water, the phase velocities of 330 m/s and 1500 m/s, respectively, give a relative index of refraction of 4.55, which means that the angle of incidence for total reflection is only 13°. As the angle of incidence approaches 90°, the angular wave impedance approaches infinity, so that B = -A, approximately. There is again total reflection, but in this case the phase of the reflected wave is reversed, as in a dense-to-rare reflection, and C = 0. If ρ'c'/cos θ' = ρc/cos θ there will be no reflected wave. Using (1/c')sin θ' = (1/c)sin θ, we can eliminate cos θ' from this relation, and find sin θ = (c/c'){[1 - (rho;'c'/ρc)2]/[1 - (ρ'/ρ)2]}1/2. A physical angle θ will exist if the quantity inside the curly brackets is greater than zero. If this is so, then waves incident at this angle will be totally transmitted. This means, of course, that the normal velocity of the incident wave equals the normal velocity of the refracted wave, so that no reflected wave is necessary. The reflection of sound from solids is a rather complex study, because of the variety of surfaces and the existence of transverse waves in solids. Bulk longitudinal waves in solids are a little faster than longitudinal waves in fluids, because of the additional shear forces. Near normal incidence, shear waves will not be excited, and our equations can be used if the proper parameters are inserted. Solid surfaces may be porous, and offer considerable dissipative resistance, as in sound-absorbing wall coverings. A solid surface may be characterized by its specific surface impedance z, the ratio of pressure to normal velocity. Applying the boundary conditions for an incident wave A and a reflected wave B, we have A + B = C and (A - B)(cos θ/ρc) = C/z. Then, B = [(z - ρc/cos θ)/(z + ρc/cos θ)] A. In general, z can be complex, z = r + jx, with r and x functions of frequency. Let β = z/ρc. The energy reflection coefficient α = |B/A|2 = [(β - 1)/(β + 1)]2, or β = (1 = √α)/(1 + √α). Assuming that z is real, an absorption coefficient of 0.5 corresponds to β = 0.172 or z = 7.4 g/s-cm2. A z of 43 g/s-cm2 would mean α = 1, or perfect absorption. Surface impedances can be measured by experiments analogous to electromagnetic transmission line experments, using the Smith chart and the standing-wave ratio. It is easy to see that for oblique incidence, the reflectivity becomes greater, and the absorption less. At glancing incidence, the surface absorption vanishes. Sound is absorbed by viscous friction at surfaces, so a sound absorber must present a large amount of surface. A permeable material is required, such as glass wool or cotton wool, and the incident sound must directly contact a permeable surface. Foamed plastics are quite unsuitable as sound absorbers, though their lightness may encourage transmission. An absorbing surface of this type is equivalent to the absorbing resistance in parallel with an acoustic inductance, which short-circuits the resistance at low frequencies. Above 1 kHz, a porous surface may have α = 0.9, but the absorption drops at low frequencies. What is usually called "styrofoam" usually isn't. Styrofoam is extruded polystyrene (XPS) made by Dow Chemical, and is blue. It has no porosity and is an excellent thermal insulator for moderate temperatures. "Styrofoam" cups and such are made from expanded polystyrene (EPS), which consists of small spheres fused together, which are quite evident on close inspection. A sample I tested had a density of 14 kg/m3 and a Young's modulus of about 500 psi or 3.5 GPa. If Poisson's ratio was about zero, then the velocity of longitudinal waves would be about 500 m/s, and the specific acoustic impedance 700 g/s-cm2. A Poisson's ratio of 1/3 would raise the phase velocity only to about 600 m/s. Neglecting the effect of any porosity, the absorption coefficient for normal incidence would be 0.22 on the basis of transmission alone. Intensity reflected from the back surface should be taken into consideration. A membrane absorber is a volume between two membranes with some kind of absorber, perhaps at the circumference. An example is a double-glazed window, but the idea here is more to reduce transmission than to absorb incident sound. This device acts like an absorbing resistance in parallel with an acoustic capacitance (and with an acoustic inductance in series, representing the mass of the membrane), absorbing best at low frequencies, but with α as high as 0.5 below about 300 Hz. A final type of absorber is the Helmholtz resonator with an absorbing plug in its neck. This is a resonant absorber, which is effective only over a narrow frequency range, and which is applicable only in special circumstances. The reverberation time of a room of volume V m3, which is the time for a sound to drop 60 dB from an initial value, is roughly given by Sabine's formula, t = 0.16V/A seconds, where A is the absorbing area in m2 times the value of α, summed over all absorbing area in the room. It is clear that large rooms require some help in absorption if the reverberation time is not to increase above a certain maximum, which depends on the size and use of the room. Small rooms should have reverberation times of around 1 second, which is the boundary between "dead" and "live" rooms. Most acoustical wave phenomena have analogies in optics, and vice-versa, except, of course, for polarization phenomena. The existence of polarization in light retarded the acceptance of the wave theory of light, and put the analogies with sound in doubt. The analogies in interference and diffraction are particulary interesting. A single sheet of cloth reflects very little sound, since the cloth is porous. However, if parallel sheets are a half-wavelength apart, the weak reflections will all be in phase, and the reflection will become strong. The action is similar to a multiple-layer reflective coating in optics. Wind and WavesWhen considering the Doppler effect, we assumed that the waves always moved with the phase velocity c in the air, independently of the movement of the source. When a wind blows, the waves are carried with the air, convected. It is a common observation that sounds can be heard better downwind of the source than upwind, or that 'the wind carries the sound.' Indeed it does, but this cannot be the explanation for this observation, since wind speeds are always much less than the speed of sound, usually less than a twentieth of the sound speed of 750 mph. In fact, a constant wind will have very little effect on the propagation of sound, not even affecting the frequency heard in different directions. However, when a wind blows, it is retarded at the surface--a sort of boundary layer effect--and increases in speed aloft. This is a wind shear, that can be expressed by a gradient dU/dy, where U is the wind speed and y is the height. A wavefront propagating with the wind will have its top inclined forward, so it will tend to return to the surface, while a wavefront propagating against the wind will be deflected upwards. This is a much better explanation of the fact that sounds can be heard better downwind than upwind.
Our analysis is based on the constancy of the trace velocity on horizontal planes, as in the case of temperature, which the present analysis includes. This can be expressed in terms of the ray inclination, making it possible to trace the paths of rays, which are the directions of energy propagation. The result is quite simple, a quadratic equation for sec &psi, that reduces to our previous result if U = 0. We notice (with Rayleigh) that sec φ = 1 corresponds to the boundary for total reflection, and the constant in this case is just c' + U', where the primed quantities are the values at the height of total reflection. This means that rays originating at the ground, assuming U = 0 there, will return to the ground if their initial inclination is less than φ, where sec φ = (c' + U')/c. Therefore, winds high in the atmosphere do affect long-distance propagation of sound, as we have assumed earlier. The radius of curvature of the rays can be found as shown in the Figure, assuming that c is constant. (If it is not, the algebra becomes much more difficult, but can be carried out in principle). The radius of curvature R reverses if the sign of the wind shear reverses, and, of course, when the direction of propagation changes. We have been cavalier with the signs here, but they can be worked out easily if you are bothered. Radiation, Scattering and DiffractionA large number of interesting problems involve waves that are not plane, for example radiation from vibrating bodies, scattering by obstacles, and diffraction. The method of basing the analysis on the displacement becomes very inconvenient, since it is a vector, so that all three components have to be considered. The way out of this is to use a new function, the vector potential φ, whose spatial derivatives are the negative components of the velocity. Now the wave can once more be described in terms of a single quantity. If we also use harmonic waves, all the magnitudes can be related to one another as in the plane-wave case, but now some of them are vectors. In particular, the wave vector k is the wave number k times a unit vector normal to the wavefront (in the direction of the ray). Since the analysis uses vector calculus, we shall not give it here, but merely quote some interesting deductions from the analysis. Consider a small volume of any shape much less than a wavelength in size. When the wave comes by, it refuses to compress or expand as the air it replaces would do, and moreover does not move back and forth with the particle velocity. The same effect would be produced by a double source (analogous to an electric dipole) aligned along the direction of the displacement, which would radiate sound in all directions. The result is that some of the energy in the wave is scattered in all directions. As in optics, the scattered amplitude is proportional to the inverse square of the wavelength, so the scattered intensity is inversely proportional to the fourth power. This strong wavelength dependence means that shorter wavelengths are preferentially scattered by small obstacles, so the lower-frequency components in the transmitted wave are relatively stronger. A complex musical note reflected by a wood may return an octave higher, since the octave is scattered sixteen times as strongly as the base note. We are not normally aware of phenomena such as shadows and diffraction with sound as we are with light. However, there is really not much difference in the two cases, only a vast difference between the wavelength and the sizes of obstacles. The usual obstacles to sound are comparable in size to the wavelength, so diffraction is very strong and sound readily bends around obstacles. Comparable obstacles to light are so small as to be invisible to the naked eye. Sound IndoorsThe principal application of acoustics has always been to structures erected for large meetings and public performances, and the assignment to make the communication between source of sound and the audience as satisfactory as possible. Vitruvius discusses the problem at length, including the effects of the echo of the first part of a word on the intelligibility of its ending, which is rather important in Latin. He faced the difficult problems of an open amphitheatre, stone surfaces, different audience sizes, and the strength of single voices. A wall behind the actors reflected sound into the audience, while the presence of the audience decreased the reverberation time to a comfortable level. Brass resonating chambers were set in the remoter parts of a theatre to reinforce frequencies that were attenuated. Reverberation time is a very important parameter. It is very difficult to speak to a considerable audience in the open, or in a dead chamber where the walls are highly absorbing and the reverberation time short. Anechoic chambers are required for acoustic measurements, but are highly unsatisfactory for speech. A long reverberation time is equally unpleasant, as earlier sounds intefere with later ones. Rayleigh noticed that the Baptistery in Pisa had a reverberation time of about 12 seconds. The notes of a common chord, sounded one after the other, are heard together for an extended time. The ear appreciates a comfortable intermediate value, such as is commonly reached in the furnished rooms of houses. Before the furniture has been brought in, the empty rooms are characteristically live. Many performance halls have been found unsatisfactory on acoustic grounds, from unwanted resonances or focussing, improper reverberation time, and badly directed sound. Few are now built without extensive acoustic design, which can be effective if well-done. When a sound wave strikes a solid surface, any velocity components along the surface are resisted by viscosity, and fluid motions in any pores or cracks in the surface are also subject to the same dissipative forces. A boundary layer of a thickness about h = sqrt(8π2ν/f) governs these phenomena. Here, f is the frequency, and ν is the kinematic viscosity, as above. The phase velocity of waves propagating down a tube of radius d (much greater than h) is reduced by hc/4πd, and the linear absorption coefficient is α = fh/4πdc. The asorption is much greater than for waves propagating in the open. When d becomes smaller than h, the motion is dominated by viscosity, and inertia has little influence. Waves are rapidly stifled in such pores. Wall hangings, carpets and acoustic ceiling tiles are, for this reason, quite effective in reducing echoes. Only in this way can sound die out in an enclosed space. The sound absorption coefficient of a surface is α = 1 - r, where r is the energy reflection coefficient. This can be evaluated for diffuse incident sound, so that it is applicable to buildings. α at frequencies of 125 Hz, 1000 Hz, and 4000 Hz for several surfaces are: acoustic tile, 0.10, 0.75, 0.50; brick, 0.02, 0.04, 0.07; oak panelling on 1" battens, 0.20, 0.05, 0.05; and snow, 0.15, 0.75, 0.85.
Applications of AcousticsThis paper has been limited to the fundamental properties of sound waves, and some of the lore directly related to this subject. The theory can be immediately extended to other than plane waves, and the important subjects of radiation and scattering can be taken up. This requires a recasting more along the lines of fluid mechanics, since using the displacement as a fundamental quantity becomes quite inconvenient in more general investigations, where potentials are more convenient. The science of sound also usually includes the treatment of the vibrations of solid bodies, which is again quite mathematical and incidentally, rather successful. Waves in solids is a distinct field with its own applications, such as seismometry, and which also plays an important role in ultrasonics. Ultrasonics is an applied branch of acoustics concerned with the production and use of frequencies above about 40 kHz, which are used for testing, measurement, ranging, communication and materials processing. Engineering acoustics is concerned with the production and measurement of sound, especially random sound or noise, and the acoustical properties of materials. Architectural acoustics studies the acoustic properties of buildings, as mentioned above. The auditory sense, or physiological acoustics, is another branch of study, whose most famous and successful investigator was Hermann von Helmholtz (1821-1894). His classic work is referenced below. Note on UnitsI have chiefly used cgs units above, following Lamb and the earlier practice. SI units differ only by powers of 10 from these, so conversion is very easy. All consistent systems of units have some that are of inconvenient size, and SI excels in this. Here is a conversion chart to help:
Standard
acceleration of gravity g = 980.665 cm/s2 References:References 1-3 are classics that should be known, if not familiar, to anyone interested in this field. There is a large number of works on acoustics, hearing and vibration to be found in any good scientific library. Some are valuable and will reward study.
Composed
by J. B.
Calvert
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||


 The
force on a thin sheet of gas of thickness dx is the
difference in the pressures
on the two sides of the sheet. This must equal the
mass of the sheet times its
acceleration, by Newton's Second Law. Since we know
the difference in the
condensation on the two sides of the sheet, which will
be the second derivative
of the displacement with respect to x, times the
thickness dx, we will know the
pressure difference if we know how condensation is
related to pressure. In an
ideal gas, the pressure is proportional to the density
when the temperature is
held constant, so in this case the fractional change
in pressure will be equal
to the fractional change in density, or the
condensation. If we let p denote the
change in pressure, then p = p0s. The
pressure variations in a sound
wave are so rapid, however, that there is no time to
reach isothermal
conditions. What occurs is an adiabatic
process in which the pressure
difference is greater due to the heating or cooling of
the gas as it is
compressed or expanded. In an adiabatic process, p =
γp0s
instead. γ is the ratio of the specific heat at
constant volume to the
specific heat at constant pressure. This was a problem
that baffled Newton, when
the calculated and measured speeds of sound did not
agree, and was only settled
later by Laplace.
The
force on a thin sheet of gas of thickness dx is the
difference in the pressures
on the two sides of the sheet. This must equal the
mass of the sheet times its
acceleration, by Newton's Second Law. Since we know
the difference in the
condensation on the two sides of the sheet, which will
be the second derivative
of the displacement with respect to x, times the
thickness dx, we will know the
pressure difference if we know how condensation is
related to pressure. In an
ideal gas, the pressure is proportional to the density
when the temperature is
held constant, so in this case the fractional change
in pressure will be equal
to the fractional change in density, or the
condensation. If we let p denote the
change in pressure, then p = p0s. The
pressure variations in a sound
wave are so rapid, however, that there is no time to
reach isothermal
conditions. What occurs is an adiabatic
process in which the pressure
difference is greater due to the heating or cooling of
the gas as it is
compressed or expanded. In an adiabatic process, p =
γp0s
instead. γ is the ratio of the specific heat at
constant volume to the
specific heat at constant pressure. This was a problem
that baffled Newton, when
the calculated and measured speeds of sound did not
agree, and was only settled
later by Laplace.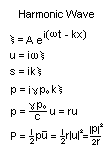 A harmonic
plane
wave traveling towards positive x has a displacement
ξ = A exp[i(ωt -
kx)]. If it is traveling in the other direction,
simply change the sign of k.
The phase velocity c = ω/k, ω = 2πf and k = 2π/λ. Now
the time derivative is the same as multiplication by
iω and the space
derivative is the same as multiplication by -ik. Now
all the quantities in the
wave are algebraically related, as shown at the right.
Note that the
overpressure and condensation are in phase, and lead
the displacement by 90°
for a wave travelling towards +x, but lag by 90° for a
wave in the opposite
direction. The particle velocity always leads the
displacement by 90°. The
power in the wave is the product of the overpressure p
and the particle velocity
u. If these are expressed as phasors, then the average
power is half the product
of one phasor times the complex conjugate of the
other, just as in alternating
current circuits. In fact, the ratio of the pressure
(potential) to the particle
velocity (current) is a quantity r = γp0/c
analogous to a wave
resistance, and is called the specific acoustic
impedance, or wave
impedance. For air, r = 42.6 dyne-sec/cm3,
and for water, 14
800.0 dyne-sec/cm3. The specific acoustic
impedance is also the
product of the density and the phase velocity. Its
units can also be expressed
as g/s-cm2 in cgs, or kg/s-m2
MKS. There has been some
effort to name this unit the rayl in honor of
Rayleigh, but this has
apparently not met with much enthusiasm.
A harmonic
plane
wave traveling towards positive x has a displacement
ξ = A exp[i(ωt -
kx)]. If it is traveling in the other direction,
simply change the sign of k.
The phase velocity c = ω/k, ω = 2πf and k = 2π/λ. Now
the time derivative is the same as multiplication by
iω and the space
derivative is the same as multiplication by -ik. Now
all the quantities in the
wave are algebraically related, as shown at the right.
Note that the
overpressure and condensation are in phase, and lead
the displacement by 90°
for a wave travelling towards +x, but lag by 90° for a
wave in the opposite
direction. The particle velocity always leads the
displacement by 90°. The
power in the wave is the product of the overpressure p
and the particle velocity
u. If these are expressed as phasors, then the average
power is half the product
of one phasor times the complex conjugate of the
other, just as in alternating
current circuits. In fact, the ratio of the pressure
(potential) to the particle
velocity (current) is a quantity r = γp0/c
analogous to a wave
resistance, and is called the specific acoustic
impedance, or wave
impedance. For air, r = 42.6 dyne-sec/cm3,
and for water, 14
800.0 dyne-sec/cm3. The specific acoustic
impedance is also the
product of the density and the phase velocity. Its
units can also be expressed
as g/s-cm2 in cgs, or kg/s-m2
MKS. There has been some
effort to name this unit the rayl in honor of
Rayleigh, but this has
apparently not met with much enthusiasm.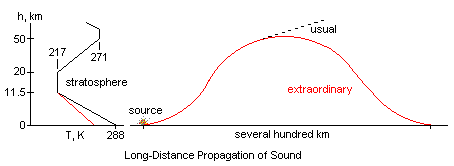 A plane wave
propagating in a thermally stratified atmosphere can
be represented by a ray
normal to the wave front whose inclination to the
horizontal changes so that the
velocity with which the line of intersection of the
wave front with a horizontal
plane moves, called the trace velocity, is constant.
If θ is the
inclination, and c(h) the phase velocity as a
function of altitude, then c(h)
sec θ = c(0) = 340 m/s, if the wave started
horizontally at the surface. As
c(h) decreases, θ increases, and the wave climbs. At
about 11.5 km, the
stratosphere is reached, where c = 295 m/s. The
inclination of the ray remains
at about 30° through the stratosphere. At 20 km, the
temperature begins to
climb again (due to absorption of solar radiation by
ozone), and reaches a
maximum at about 49 km, where c = 330 m/s. The ray
bends over, and at the
maximum is inclined only 14° with the horizontal.
Should the temperature up
here be a little hotter than normal, and the
temperature on the ground a little
colder than normal, or a strong wind shear in the
direction of propagation exist
on high, or else a strong inversion exist on the
ground, the ray will become
horizontal, and then follow the mirror of its
previous path down to the ground
again, about 200km from the source. At 50 km, the
mean free path of the air
molecules is still no more than 0.1 mm, so the wave
will not be strongly
absorbed, especially at lower frequencies. The
effect is analogous to the
reflection of radio waves by the ionosphere, which
also show 'skip' phenomena.
Wind directions favour east to west propagation in
the summer in northern
temperate latitudes, and make it extremely unlikely
in the winter.
A plane wave
propagating in a thermally stratified atmosphere can
be represented by a ray
normal to the wave front whose inclination to the
horizontal changes so that the
velocity with which the line of intersection of the
wave front with a horizontal
plane moves, called the trace velocity, is constant.
If θ is the
inclination, and c(h) the phase velocity as a
function of altitude, then c(h)
sec θ = c(0) = 340 m/s, if the wave started
horizontally at the surface. As
c(h) decreases, θ increases, and the wave climbs. At
about 11.5 km, the
stratosphere is reached, where c = 295 m/s. The
inclination of the ray remains
at about 30° through the stratosphere. At 20 km, the
temperature begins to
climb again (due to absorption of solar radiation by
ozone), and reaches a
maximum at about 49 km, where c = 330 m/s. The ray
bends over, and at the
maximum is inclined only 14° with the horizontal.
Should the temperature up
here be a little hotter than normal, and the
temperature on the ground a little
colder than normal, or a strong wind shear in the
direction of propagation exist
on high, or else a strong inversion exist on the
ground, the ray will become
horizontal, and then follow the mirror of its
previous path down to the ground
again, about 200km from the source. At 50 km, the
mean free path of the air
molecules is still no more than 0.1 mm, so the wave
will not be strongly
absorbed, especially at lower frequencies. The
effect is analogous to the
reflection of radio waves by the ionosphere, which
also show 'skip' phenomena.
Wind directions favour east to west propagation in
the summer in northern
temperate latitudes, and make it extremely unlikely
in the winter.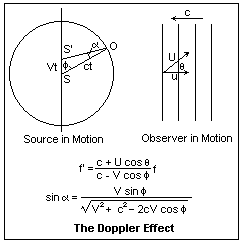 A purely
geometrical effect conceived by Christian Doppler in
1842 is the apparent
increase in frequency of sound when the source and
observer are approaching, and
the decrease of frequency when they are receding. He
apparently thought of it
with respect to the light from double stars, as a red
or blue shift. This was
ignored in acoustics until railways finally gave
speeds high enough relative to
the speed of sound to make the effect noticeable.
Buijs Ballot put musicians on
an open carriage pushed by a locomotive at various
speeds in 1845, and measured
the change in frequency. Scott Russell, the naval
architect, also investigated
the effect. Mach studied it with a rotating whistle in
1861 . Sound propagates
through the medium with a speed independent of the
motions of source and
observer, of course. The motions of both source and
observer relative to the
medium is what is important, not simply their motions
relative to one another,
as in the case of light. There is also aberration,
or an apparent change
in direction, when the source and observer are not
moving directly toward each
other or apart. This is easily noticed with low-flying
jet aircraft, where the
sound appears to come from behind the aircraft. The
Figure shows the wavefront
emitted by a source at S just reaching the observer O,
at which time the source
is actually at S'. If a wind is blowing, but the
source and observer are
stationary, then there is no change in frequency of
the observed sound.
A purely
geometrical effect conceived by Christian Doppler in
1842 is the apparent
increase in frequency of sound when the source and
observer are approaching, and
the decrease of frequency when they are receding. He
apparently thought of it
with respect to the light from double stars, as a red
or blue shift. This was
ignored in acoustics until railways finally gave
speeds high enough relative to
the speed of sound to make the effect noticeable.
Buijs Ballot put musicians on
an open carriage pushed by a locomotive at various
speeds in 1845, and measured
the change in frequency. Scott Russell, the naval
architect, also investigated
the effect. Mach studied it with a rotating whistle in
1861 . Sound propagates
through the medium with a speed independent of the
motions of source and
observer, of course. The motions of both source and
observer relative to the
medium is what is important, not simply their motions
relative to one another,
as in the case of light. There is also aberration,
or an apparent change
in direction, when the source and observer are not
moving directly toward each
other or apart. This is easily noticed with low-flying
jet aircraft, where the
sound appears to come from behind the aircraft. The
Figure shows the wavefront
emitted by a source at S just reaching the observer O,
at which time the source
is actually at S'. If a wind is blowing, but the
source and observer are
stationary, then there is no change in frequency of
the observed sound.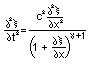 Our analysis is
most likely to be found inadequate in practice with
very strong sound waves. A
more accurate wave equation, in which the condensation
is not neglected compared
to unity, is shown at the left. Note that (1 + s) now
appears in the denominator
on the right-hand side. Since this equation is
nonlinear, its solutions will not
obey the principle of superposition, and the shape of
a wave will change as it
moves. Analysis of this equation shows that the phase
velocity is slightly
greater where the condensation is greater.
Essentially, the particle velocity is
added to the phase velocity. The more condensed
portions of the wave move
forward relatively to the less condensed, and the
front of the wave becomes
steeper. Such behaviour is familiar from water waves,
which are also nonlinear
in shallow water (as well as being generally
dispersive). Nonlinearity also
results in the production of sum and difference tones,
and other new
frequencies, when waves are superimposed. Should the
front of a wave become so
steep that it becomes a discontinuity, or shock
wave, viscosity and heat
conduction become essential to the analysis. By the
action of spreading and
dissipative processes, the shock wave can again become
a continuous sound wave.
In nearly all cases except those dealing with
explosions and shock waves,
nonlinear effects in the propagation of sound waves
are completely negligible.
Our analysis is
most likely to be found inadequate in practice with
very strong sound waves. A
more accurate wave equation, in which the condensation
is not neglected compared
to unity, is shown at the left. Note that (1 + s) now
appears in the denominator
on the right-hand side. Since this equation is
nonlinear, its solutions will not
obey the principle of superposition, and the shape of
a wave will change as it
moves. Analysis of this equation shows that the phase
velocity is slightly
greater where the condensation is greater.
Essentially, the particle velocity is
added to the phase velocity. The more condensed
portions of the wave move
forward relatively to the less condensed, and the
front of the wave becomes
steeper. Such behaviour is familiar from water waves,
which are also nonlinear
in shallow water (as well as being generally
dispersive). Nonlinearity also
results in the production of sum and difference tones,
and other new
frequencies, when waves are superimposed. Should the
front of a wave become so
steep that it becomes a discontinuity, or shock
wave, viscosity and heat
conduction become essential to the analysis. By the
action of spreading and
dissipative processes, the shock wave can again become
a continuous sound wave.
In nearly all cases except those dealing with
explosions and shock waves,
nonlinear effects in the propagation of sound waves
are completely negligible. When
a plane sound wave encounters a plane material
interface, it is reflected and
refracted according to the laws familiar from optics.
The amounts reflected and
transmitted depend on the relative acoustic impedance
of the two media. We can
easily solve the problem for normal incidence by using
the results we have
already found. In the figure, quantities in the
incident wave are unprimed,
those in the reflected wave singly primed, and those
in the transmitted wave are
doubly primed. The waves are described by their
displacements ξ. The volume
elasticity κ is used for generality, instead of γp for
an ideal gas.
At the boundary, the two media must remain in contact
as the wave passes, and
the pressures must be equal on the two sides of the
interface. These two
boundary conditions can be expressed as shown in terms
of the displacements. The
pressure condition is put in terms of the displacement
by assuming a harmonic
wave, a very convenient, but not essential, way to
proceed here. Everything can
be expressed in terms of the acoustic impedances r and
r" of the two media.
Finally, the simultaneous equations are easily solved
for ξ' and ξ"
in terms of ξ, and the results appear in the box.
When
a plane sound wave encounters a plane material
interface, it is reflected and
refracted according to the laws familiar from optics.
The amounts reflected and
transmitted depend on the relative acoustic impedance
of the two media. We can
easily solve the problem for normal incidence by using
the results we have
already found. In the figure, quantities in the
incident wave are unprimed,
those in the reflected wave singly primed, and those
in the transmitted wave are
doubly primed. The waves are described by their
displacements ξ. The volume
elasticity κ is used for generality, instead of γp for
an ideal gas.
At the boundary, the two media must remain in contact
as the wave passes, and
the pressures must be equal on the two sides of the
interface. These two
boundary conditions can be expressed as shown in terms
of the displacements. The
pressure condition is put in terms of the displacement
by assuming a harmonic
wave, a very convenient, but not essential, way to
proceed here. Everything can
be expressed in terms of the acoustic impedances r and
r" of the two media.
Finally, the simultaneous equations are easily solved
for ξ' and ξ"
in terms of ξ, and the results appear in the box.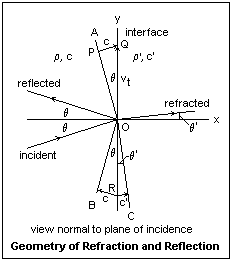 The
figure shows the wavefronts at the instant of time
when the trace is at the
origin O. The x-coordinate is normal to the interface,
the y-coordinate in the
interface, and the z-coordinate normal to both. The
z-axis is the common trace
of the three wavefronts. The incident wave is given by
Ae-jk(x cos θ +
y sin θ), the reflected wave by Bejk(x
cos θ - y sin θ),
and the refracted wave by Ce-jk'(x cos θ' + y sin
θ'). The
wave vector k' for x > 0 is given by k' = ω/c'.
Note that ω is the
same for all the waves involved. On the interface, x =
0, so the waves are Ae-jyk
sin θ, Be-jyk sin θ and Ce-jk'
sin θ'.
We have already found that k sin θ = k' sin θ', so the
exponential
factors giving the y-dependence are all the same. We
come to the important
conclusion, then, that if the boundary conditions are
satisified at one point on
the interface, then they are satisfied at all points
on the interface.
The
figure shows the wavefronts at the instant of time
when the trace is at the
origin O. The x-coordinate is normal to the interface,
the y-coordinate in the
interface, and the z-coordinate normal to both. The
z-axis is the common trace
of the three wavefronts. The incident wave is given by
Ae-jk(x cos θ +
y sin θ), the reflected wave by Bejk(x
cos θ - y sin θ),
and the refracted wave by Ce-jk'(x cos θ' + y sin
θ'). The
wave vector k' for x > 0 is given by k' = ω/c'.
Note that ω is the
same for all the waves involved. On the interface, x =
0, so the waves are Ae-jyk
sin θ, Be-jyk sin θ and Ce-jk'
sin θ'.
We have already found that k sin θ = k' sin θ', so the
exponential
factors giving the y-dependence are all the same. We
come to the important
conclusion, then, that if the boundary conditions are
satisified at one point on
the interface, then they are satisfied at all points
on the interface.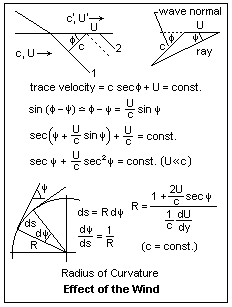 Let us
consider the
case of a horizontally stratified atmosphere with
temperature and wind varying
with altitude, and a plane wavefront propagating with
its normal making an angle
φ with the horizontal. Note that this is a different
convention than we
used above in considering the effect of temperature,
where we used the
convention familiar from optics. The wind U convects
the wavefront, so that the
rays are no longer normal to the wavefronts, but
deviate slightly in the
direction of the wind. Let the inclination of the ray
with the horizontal be
ψ. The Figure at the right shows how φ and ψ are
related. We can
use the fact that U is much less than c to simplify
the formulas. We assume
nothing regarding the magnitude of φ or ψ.
Let us
consider the
case of a horizontally stratified atmosphere with
temperature and wind varying
with altitude, and a plane wavefront propagating with
its normal making an angle
φ with the horizontal. Note that this is a different
convention than we
used above in considering the effect of temperature,
where we used the
convention familiar from optics. The wind U convects
the wavefront, so that the
rays are no longer normal to the wavefronts, but
deviate slightly in the
direction of the wind. Let the inclination of the ray
with the horizontal be
ψ. The Figure at the right shows how φ and ψ are
related. We can
use the fact that U is much less than c to simplify
the formulas. We assume
nothing regarding the magnitude of φ or ψ. In the
gallery
around the base of the dome in St Paul's cathedral in
London, it has long been
noticed that the voices of people on the other side of
the dome are easily heard
near the wall. This is an example of a whispering
gallery, of which
others are known. Some have regarded the abnormal
audibility as due to the
focusing effect of the spherical surface. Indeed,
sound issuing from one focus
of an ellipsoid will be directed towards the other
focus, and sound from one end
of the diameter of a circle may be intensified at the
other end of the diameter,
which lies on a caustic surface. Sir George Airy
thought this the explanation of
St Paul's whispering gallery. Lord Rayleigh, on the
other hand, has given a
plausible explanation that is more generally
applicable. The sound emitted in a
solid angle along a tangent to the curved wall is
restricted to lie within a
certain spherical shell, as indicated in the Figure,
so its intensity will
decrease with spreading only as 1/r, not as 1/r2.
Therefore, sound
tends to cling to a concave wall in general.
In the
gallery
around the base of the dome in St Paul's cathedral in
London, it has long been
noticed that the voices of people on the other side of
the dome are easily heard
near the wall. This is an example of a whispering
gallery, of which
others are known. Some have regarded the abnormal
audibility as due to the
focusing effect of the spherical surface. Indeed,
sound issuing from one focus
of an ellipsoid will be directed towards the other
focus, and sound from one end
of the diameter of a circle may be intensified at the
other end of the diameter,
which lies on a caustic surface. Sir George Airy
thought this the explanation of
St Paul's whispering gallery. Lord Rayleigh, on the
other hand, has given a
plausible explanation that is more generally
applicable. The sound emitted in a
solid angle along a tangent to the curved wall is
restricted to lie within a
certain spherical shell, as indicated in the Figure,
so its intensity will
decrease with spreading only as 1/r, not as 1/r2.
Therefore, sound
tends to cling to a concave wall in general.